Discussion Topic: Reading Discussion 4
Read the excerpt from the Standards Decoded document. This is a description of all the standards in the Ratio and Proportion domain.
Respond to the following questions:
1. In a “traditional” curriculum, the topic of proportion was often limited to the “cross-multiply and divide” algorithm. What are three ways in which the CCSS approach to developing proportional reasoning differs from the more “traditional” algorithmic approach? In what ways would this approach to teaching proportional reasoning benefit students (e.g., in terms of connections to future mathematics learning)?
2. The Standards Decoded document is one resource that we will examine in the course that provides additional information for understanding or “decoding” the standards. Describe one idea from this document that was important to your own “decoding” of the RP domain. Why was this idea significant?
6.RP.A*
E
‘F
E
t
t
E
t
E
E
E
E
E
r
r
I
I
I
I
T
I
I
6.RP.A.1: Uriderstand the concept of a ratio and-use ratio language to describe a ratio relationship
betweentwoquantities.i;-r;;;ib,-“Tle..rg!ooliingtnbe&.s.iithebirdhouseatthezoorlasl:l’
because for every 2 wings tn l, i”i |’beak.” “Fo, nnury iotu candidate Areceived’ candidate C receited
nearly three votes.”
5.RPA.2: Understand the concept of a unit rate a/b associated with a ratio a:b with b + 0, and
use rate language in tfr” .o”t.*i t’f , ,atio relationship. For example, “This recipe has a ratio of 3
cups of flour to 4 cups of sugar, so there is 1o cup of flour for each cup of sugar'” “we paid $7 5 for 1>
hamburgers, which is a rate of $5 per hamburger'”
\
rExpectations for unit rates in this grade are limited to non-complex fractions’
6.Rp.A.3: Use ratio and rate reasoning to solve real-world and mathematical Problems, e’g’,
;;;;–ril;t;;;irbt”; ;i”quivaleniratios, tape diagrams, double number line diagrams, or
equations
.
a. Make tables of equivalent ratios relating quantities with whole-number measurements’ find
missing values in,f,. ,rif* ,”a plot th”e pri* of urtu.r on the coordinate plane’ Use tables to
comPare ratios’
b. Solve unit rate problems including those involving unit pricing and constant speed’ For
example, yittooni iourc io *o*4 to*n ,iin, oithotritn, hiw *ary lawns couldbe mowed
in 35′ horurs? At what rate were lawns being mowed?
c. Find a percent of a quantity as a rate per I00 (e’g”)0%of a quantity *t”‘ ffi times the
quantity); solve problems involving finding the whole’ given a part and the percen
t’
d.Useratioreasoningtoconvertmeasurementunits;manipulateandtransformunits
apfropriately whei multiplying or dividing quantities’
oMajor cluster
cluster A: understand ratio concepts and use ratio reasoning to solve problems’
Grade 5 Overview
The focus for this cluster is the study of ratio concepts and the use of proportional reasoning to solve oroblems’ Students learn
how ratios and rates are used to compare t*o q.,rr,’tnni.l;;;;i;;ffi’h;;;’;;”iilffi’;;;;,.thim’ Sixth graders find or’rt
how ratios are used i, ,”;i-;Ji;ir-riior, ,.,d dir”ou., solutions to p-“”””i pioblems using ratio tables’ tape diagrams’ and
doublenumberlines.Studentsalsoconvertbetweenstandardunitsofmeasure.
Standards for Mathematical Practice
SFMP 1. Make sense of problems and persevere in solving them’
Sixth graders interpret and solve ratio problems’
t 6 The common core Mathematics companion: The Standards Decoded’ Grades 6-8
In this standard, students learn to compare two quantities or measures such as 6:l or 10:2. These compafisons are called ratios.
Students discover that ratios can be written and described in different ways. For instance, 6:l uses a colon to separate values.
Ratios can also be stated with words such as 6 to l, or as a fraction such as -6. . Standard I focuses on understanding the concept
of a ratio, however, students should use ratio language to describe real-world experiences and use their understanding for decision
making.
ot
Help students discover that a ratio is a relationship or
comparison of two quantities or measures. Ratios compare
two rneasures of the same types of things such as the
number of one color of socks to another color of socks or
two different things such as the number of squirrels to birds
in the park. Ratios compare parts to a whole (part:whole)
such as l0 of our 25 students take music lessons. Ratios
can also compare a part of one whole to another part of
the same whole (part:part) such as the ratio of white socks
in the drawer to black socks in the drawer is 4:6. Ratios are
expressed or written as d to b, a:b, or t
Compare and model ratios with real-world things such as
pants to shirts or hot dogs to buns. Ratios can be stated as
the comparison of 10 pairs of pants to I B shirts and can
be written as l-0, I0 to IB, or 10:18 and simplified to,
-B
;, 5 to 9, or 5:9. Ensure that students understand how
the simplified values relate to the original numbers.
Ask students to create or find simple real-world problems
to use in their learning such as, “There dre 2 Thoroughbred
horses and 6 Appaloosa horses in the field. As a ratio of
Thoroughbreds to Appaloosas it is: Z or 2 to 6 or 2:6 or
6
simplified as L, I to 3, or 7:3. Or, there are 14 girls and 18
5
boys in our math class. As a ratio of girls to boys it is: # ,
]B
14 to 18, ar 14:18 or simplified as 7-^ ,7 to 9, or 7:9.” Invite
9
students to share their real-world examples of ratios and
use ratio language to describe their findings such as, “for
every yote candidate A receiyed, candidate C received nearly
three votes.” The problems students select or write can
also be used as cyclical reviews with distributed practice
throughout the school year.
Focus on the following vocabulary terms: ratio, compare,
and simplifu.
Understand that a ratio is a comparison between quantities.
Determine when a ratio is describing part-to-part or part-to-
whole comparison.
o Describe ratio relationships between two quantities using
ratio language.
o Use the different ratio formats interchangeably
(4:5,4 to 5, I
l
)
Some sixth graders may confuse the order of the quantities such as when asked to write the ratio of boys to g_irls in the- sentence,
“There are 1-4 girls and 18 boys in our math c/ass.” Instead of writing l8: 14, some 5fudents may write 14: 18. Other students may
not recognize-th” diff.r.rr.. between a part-to-part ratio and a part-to-whole ratio such as,”There are 14-gi-*.gynpared to 18
boys in tte class (14:18 part-to-part); howewr, 14 of the 32 students in our class are girls Qa32 pyt19-whole).” To address these
cornmon misconceptions, ask students to label the quantities they are comparing such as 14 girls/I8 boys.
8 The Common Core Mathematics Companion: The Standards Decoded, Grades 5-8
a
–
H
–
H
Ea
i ‘:siations for unit rates in this grade are limited to non-complex fractions.
T-:s standard focuses student learning on the concept of a unit rate as a special kind of ratio. Students compare different units
:,: n.ieasure such as the amount of rhoney earned to the hours worked while babysitting and calculate unit rates by setting up
:atios and simplifying them. Students understand a situation in ratio form and write the unit that describes the situation using
appropriate rate language with words such as per and symbols such as / to compare different units or measures.
flfl
Begin by exploring the difference between a ratio and a
rate. Rate is a special ratio that compares two quantities
with different units of measure. Share multiple examples
for students to make sense of the concept for rate such as,
“LaShanda babysat for $:S for 7 hours.” Or,’oDad\ new
truck got 400 miles on 20 gallons of gas.” Then explore
the unit rate that expresses a ratio as part-to-one. Generate
examples such as “Lashanda is paid a unit rdte of $5 per 1
hour for babysitting (5:1)” and “My dad’s new truck gets 20
miles per gallon of gas (20:1).”
Ask students to locate and share real-world examples of
cost per item or distance per time in newspapers, ads, or
other media. (Note that in sixth grade, students do not work
with unit rates expressed as complex fractions. Both the
numerator and denominator of the original ratio will be
whole numbers.)
Model how to convert rates from fraction form to word
form using per, each, or @ such as 360 milesll? gallons of
gas – 30 miles per gallon of gas. Allow students to talk with
each other and their teacher to make sense of what they are
learning and then write and share several rate conversion
examples of their own.
Focus on the following vocabulary terms: ratios, rdtes, unit
rdtes, compare, and perl@. Math journals or exit slips at
the end of a math class with writing prornpts such as, “An
example of a ratio and a problem that goes with it is.
provide closure.
Provide cyclical, distributed practice over time to
continually review simple unit rate problems.
[Jnderstand rate as a ratio that compares two quantities with
different units of measure.
l]nderstand that unit rates are the ratio of two
measurements or quantities in which the second term
means “one” such as 60 miles per one hour.
Interpret rate language with the @ symbol or with the words
per andlor eacLt.
Solve unit rate problems.
Students often confuse the terms ratio, rate, and unit rate. Try using a paper foldable with vocabulary definitions to help students
with these confusing terms. To make the foldable, divide an 8 j x I l-inch sheet of blank paper in half horizontally. Then fold
it into thirds as if a letter is being folded to fit an envelope. Unfold and write a term on each of the sections. On the inside of the
foldable, write the definitions that match each term. Students may want to cut on the vertical fold lines to flip up each section to
practice the definitions.
fold line
rate unit rate
9
ratio
fold and cut lines
Part 1 Ratios and Proportional Relationships
^a
“oblemt
use {&tioand rafe reasonins to sorve y3{-wai’! 1i,1″T:T*’Tj :’;r-rions
by reasonirtg about tables of 4
ff
i
,ii,,
C
E
l
T
.
r
I
I
t
I
l
I
I
il
ffi;ffi iiasreatioeoi@tierwith*:S’;I?::r1:i;”,:ff:::’:;i,:’missins
vinq,unit pricing and cbnstant speed’
salire unitra te p,roblems includinu,tlo:9
-1
n31r.,;J,-^ri raiirnc rould be mowed in 35
For exarnple, if
‘,,fl:1ffiflf#fffifi:l?’J|lii.31liii];”#’H;;’i#;;’;;id
b”,”owed in:s hours? Atwhat
. ;IJ:l:*;;lT# itiiw 1s
a rltlyie: 100 t”‘s’.3.Y:J::::IY **n’lfitimes the quantitv); sotve
probtems in**’^rliii”;; ‘h”;;;i;’
siven a part and r:”
,Y”n’
d, Use ratio reasoning to eanvert.measurefflent units; manipulate:and transfarm units appropriately when
” -iitiii
wng ot”dividing quantities’
In these standards, students use reasoning about murtiprication and division to solve a variety of ratio and rate problems about
cuantities. They make triil, of
“q,rivaleit
ratios r:Tii,,ru6;ili;;;’tc;ole-number *”rrur”*”r,t,, find missingvalues in the
t^bl.r, .r,d plot the 0.,*'”i”ii”.l “”ii,.'”””iarr#pi”?r.
rhey use trur”r l””””irprr” rrir”r1″a solve unit rate and constant
speed problems. Problems involving finding th” *hol” given a part and th” p.,.”.,t, such as Z0% of aquantity *.,,,, ffi , ,,”
arso a focus. For these standards, students can use equivarent ratio tabres, tape diagrams’ double number lines’ or equations’
Students connect ratios and fractions’
values in
it took
rate we re
CD Costs
What the TfACt-lER does:
. Exprore ratios and rates used in ratio tables and graphs
to solve probl”ms. Pose a ratio situation problem with
students such as”3 CD”ost $45 ‘Whatwould 8 CDs
cost? How mdny cDs-cam be purchased for $ I50’007″ To
solve the problem, students can use ratios, unit rates’ and
multiplicative ,.,’o”i”g iy 9″”1’g iid filling in the
missing values on a .f,r?t. tft.y shJuld note that if three
CDs cost $45, one Ci;;fi “ott
$15′ Every CP purchased
is an additional $1t’ $1i iiltt the number of CDs = the
cost.TheywriteanequationsuchasC-$15n.
$1 20
$1 05
$go
$75
$oo
$45
$so
$15
Investigate unit rate problems, including unit pricing
such ,r, “gli.i. Si;i h-.a, 12’iz. drinks for
has r6-oz.’-ariw rris r “rg . which drink cosrs the least per
ounce?” A;i;; students to create ratio and rate reasoning
examples to comPare and solve real-world problems’
Students could use newspapers, store ads, or online ads to
find th” .*I”-rpf.t ,r,a *Ltf the comparisons’ Ask students
to use ,.rto”i;r to determine the better buys’
. Explore finding a percent of a quanlity as a rate per 100
such as 4A%of a quantity means # times the quantity’
NotingthataPercentisarateperl00,modelhowa
(continued)
Ask students to plot the points,o” 1
coordinate plane
and draw conclisions ,bo,rt what is happening with the
pr”Ui.* ,bou.. Students should reason that for every one
movement to the right on the x-axis, the y-axis increases to
‘
15x. Also, for every one movement to the left on the x-axis’
th” Y-r*is decreases bY I 5 ‘
10 The common core Mathematics companion: The Standards Decoded, Grades 6-8
3456
Number of GDs
e
e
-{
-{
Td
IId
IIi
; =t:::.: : i: l. repre sented with a hundreds grid by
-,. -:.. ; .:,l,-, units. Have students write this as a fraction
-:
–
. a ie cinral r0.40), and a percent $A%). Consider
u:rxrE a pe rcent w’heel (see Reproducible 1) or use double
nunrber lines and tape diagrams in which the whole is 100
to find the rate per hundred.
o Solr.e problems involving finding the whole, given
a part and the percent such as, “What is 40% of 60?
80% of what number is 300? Or 50 is 30% of
what number?”
. Examine the process of how to use ratio reasoning to
convert measurement units such as, “How mdny centimeters
are in 5 feet?” Use the information that I inch = /.54 cm.
Represent the conversion of 12 inches : I ft as a conversion
lactor in ratio form, 12. ilches
.
I foot
Then multiply lZ-ilches x + = 60 inches.
I foot I
Create and interpret a table of equivalent ratios.
Plot pairs of values from a table to a coordinate plane.
Use a table to compare ratios and find missing values using
ratios.
Explain the difference betwden a ratio and a unit rate.
[Jnderstand that rate problems compare two different units,
such as revolutions per minute.
Solve real-world problems using ratios and rates.
Reason to determine the better buy.
Write a percent as a rate over 100, including percents
greater than 100 and less than I .
Find the percent of a number using rate methods.
Represent the relationship of part to whole to describe
percents using models.
Convert units by multiplication or division.
ild
T4
Then 60 inches x z’54 cm – 15 2.4 cm.
I inch
(I\ote that conversions can be made between units within a
medsurement system such as inches to feet or between systems
such as miles to centimeters.)
Allow students to talk with each other and their teacher to
make sense of what they are learning.
Focus on the following vocabulary terms: ratios, rdtes,
unit rdtes, equivalent ratios, percents, ratio tables, and tape
diagrams.
Provide cyclical, distributed practice over time to
continually practice unit rate problems.
Some sixth graders misunderstand and believe that a percent is always a natural number less than or equal to 100. To help with
this misconception, provide examples of percent amounts that are greater than 100% and percent amounts that are less than I %.
Try using a percent wheel for developing this understanding. See Reproducible 1.
T
‘It
EI
E1
ts
{
Efl–
h
!
=
h,t
b:il
b
=
E
ET
EI
i,E[-r Part 1 Ratios and Proportional Relationships 1’l
7,’nt
t’
7.RP.A*
7.RP.A.l: Compute unit rates associated with ratios of fractions, including ratios of lengths, areas
and other quantities measured in like or different :units. For example, if a person walks L mile in
each ! hour, compute the unit rate as the complex fraction Z mibs per hour, equi’talently 2 miles per
t’-Lnour. 4
7.RP.A.2: Recognize and represent proportional relationships between quantities.
a. Decide whether two quantities are in a proportional relationship, e.g., by testing for equivalent
ratios in a table or graphing on a coordinate plane and observing whether the graph is a
straight line through the origin.
b. Identify the constant of proportionality (unit rate) in tables, graphs, equations, diagrams, and
verbal descriptions of proportional
relationships.
c. Represent proportional relationships by equations. For example, if total cost t is proportional
to the number n of items purchased dt o constdnt pice p, the relationship between the total cost
and the number of items can be expressed as t = pn.
d. Explain what a point (x, y) on the graph of a proportional relationship means in terms of the
situation, with special attention to the points (0, 0) and (1, r) where r is the unit rate.
7.RP.A.3: Use proportional relationships to solve multi-step ratio and percent problems. Examples:
simple interest, tax, markups and markdowns, gratuities and commissions, fees, percent increase and
decrease, percent enor.
oMajor cluster
Cluster A: Analyze proportional relationships and use them to solve real-world and mathematical problems.
Grade 7 Overview
These standards extend what students learned in Grade 6 about ratios to analyzingproportions and proportional relationships.
Students calculate unit rates with complex fractions and move to recognizing and representing proportional relationships in
equations and on graphs. These skills and understandings are used to solve multi-step ratio and percent problems involving real-
world scenarios such as interest, tax, shopping sales, and so on.
‘ Standards for Mathematical Practice
SFMP 1. Make sense of problems and persevere in solving them.
Students solve multi-step ratio and real-world percent problems.
SFMP 3. Construct viable arguments and critique the reasoning of others.
Students recognize proportional relationships from non-proportional ones and discuss their reasoning with others.
E
t
tr
E
t
E
t
t
E
E
E
E
E
t
E
E
E
tr
E
Eh
-F16 The Common Core Mathematics Companion: The Standards Decoded, Grades 5-8
r
{crnpute r”l,tif raies
ir)s€,ts$red fn ifke *r
:1
csrnpl*x fractinn
?
e
assocrated with ratios of fractions, including ratios of lengths, areas and olher q,uantities
different units. For example, if a person walks J mile in each ] hour, compute the unit rate at the24
milesperhouLequivalently2milesperhour……i-
This standard focuses on computing unit rates using ratios of fractions known as complex fractions. In a complex fraction, the
-L
numerator, denominator, or both are fractions. In the standard, { is an example of a complex fraction. Complex fractions can
,L+
be interpreted as division statements. For example, ! “^nbe
thought of ar ] + { . Applications include situations where the
4
quantities are measured in different units such as miles per hour, pounds per square foot, feet per second, and so on.
What th* TfiACI'{ER d*es:
o Explore unit rates with ratios of fractions and compare them
to unit rates with whole numbers from Grade 6.
o Treat complex fractions as division of fractions.
. Set up error analysis scenarios where students can identify
errors in computing unit rates with complex fractions. For
example, Homer calculated that if a person walks + mile
2
every + hour, the unit rate is 2 miles. However, Homer,4
made an error. F ind his error, correct it, and explain to
Homer why 2 miles is not the correct answer.
o Provide opportunities for students to compute the unit rates
in real-world problems.
What the STUDENTS do:
o Discover that the structure of computing unit rates with
whole numbers is the same concept as unit rates with ratios
of fractions.
. Compute unit rates in real-world problems that involve
complex fractions.
. In writing, explain the errors that can be made when
computing unit rates with complex fractions and unlike
units.
Sometimes the format of a complex
I + I . Discuss how the divisionZ4
Addressing Student Misconceptions and Common Errors
It is not uncommon to find seventh-grade students who are not fluent with fraction division.
fraction confuses them when they are used to seeing fraction division written horizontally as
bar in the complex fraction means the same as the symbol +.
For students having difficulty underStanding unit rate and those having trouble with different units such as miles per hour,
pictures and diagrams may help. Use the example from this standard: lf a person walks L mile in each \ hour, compute the unit’
_L- 2 4
rate as the complex fraction t miles per hour, equivalently 2 miles per hour. Model with a diagram as shown. The bar represents
I hour broken into ] hour rlg.n.r,t .
4
mile
I
1
I
1
I
1
I
1
mile mile mile
From this diagram, students can see that the word problem is showing mile everv
J
hour.I
1
I
z
L
18 The Common Core Mathematics Companion: The Standards Decoded, Grades 6-8
L-
Fb.5
LHIHIH
EL-
I-J
E
LI
L
L-)
Sections a-d of 7.RP.A.2 break down the standard to give guidance on ways to recognize and represent proportional relationships.
This standard emphasizes hryo metliods for deciding whether a proportional relationship exists. One method is to use equivalent
ratios in a table. Ifthe ratios are equivalent, then you have a proportional relationship such as:
# of people in a room
# of hands in the room
The other method is to graph the relationship on a coordinate plane and observe whether the graph is a straight line that goes
through the origin. Note that computation using cross-multiplication is not a part of this standird.
r2345
2+68?
Explore proportional reasoning scenarios with students
to be sure they understand the meaning of proportional
relationships in context before using the tables or graphs.
While some number combinations may be proportional,
the real-world example attached to the numbers may
not be. [Jse examples and non-examples for students to
identify and compare. An example is: “2 music downloads
cost $1.98; therefore, 4 music downloads cost $3.96.” A
non-example ts: “Three boys cdn run d mile in 10 minutes;
therefore, 6 boys cdn run d mile in 20 minutes.”
Ask students to write their own examples and non-examples
of proportional relationships. Student work can be shared
and discussed.
Discuss equivalent ratios with the students. Ask them
to suggest some equivalent pairs. Relate to equivalent
fractions. Display the pairs as the students suggest them
in the form t=i, where b + }and d * 0. Define two
equivalent ratios as a proportion.
Pose examples of proportions written with the quantities in
different positions. Encourage students to decide if there
is more than one correct way to set up a proportion. For
exampl e: “Set up d proportion showing that 3 out of
15 students are girls is the sdme ratio as I out of 5 students
are girls.”
fuk students to explain how they know
proportion for the example.
is not a correct
. Craph two ratios on a coordinate plane from a proportional
scenario and look for a straight line that goes thrrough the
origin to determine if the two ratios are proportional. For
exampl e: “Mdria sells necklaces and makes a profit of $6 for
each necklace. How much monq) does she make for selling
3 neckldces?”
.(3, 18)
Pose the task to students: Select other points on the graphed
line and determine if they are also proportional.
3 1 15 5 r 5
E:’ O’T=T O’ 7:E
I
I
I
t”l
I
I
I
Ii ‘(1’ 6)
I
I
I
I
<=--- I -_-+,.
I
I
7 15or;:TI)
15 _ 2T7
Provide examples of equivalent and non-equivalent ratios
to students for them to test with a table to decide if they are
proportions. Conversely, present students with a table for a
context and ask them to determine if all of the entries in the
table are proportional.
Pa rt 1 Ratios a nd Proportiona I Relationsh ips 1 9
I
What the STUDENTS do:
. Sort real-world exampl.Ss of proportional relationships from
non-examples. Students can create their own examples
to demonstrate that they understand the concept of
proportional relationships when there is a context attached.
o Communicate orally and/or in writing that a proportion is a
statement of two equivalent ratios. Students apply what they
know about equivalent fractions to equivalent ratios”
o Model proportional relationships by creating tables;
determine if a proportional relationship exists from a given
table.
Model relationships on graphs to determine if they are
proportional.
Test their hypotheses about whether a proportional
relationship exists between any two points on the lines
graphed. Students may draw the conclusion that all points
on the line are proportional toall other points on the line
by relying on tables, verbal statements, or logic al arguments
to draw the conclusion q
E
E
l
E
c
d
T
E
Addressing Student Misconceptions and Common Errors
While graphing, students may need to be reminded that the same types of quantities need to be graphed on the same axis. For
example, when checking to determine if I0 cans of soda for $2 is proportional to 50 cans of soda for $10, the cans of soda must
both be represented on the same axis and the dollar amounts must be on the other axis. Ensure students are using graph paper or
graphing calculators for all graphing. Remind them to label the axes.
i
\
I
20 The Common Core Mathematics Companion: The Standards Decoded, Grades 5-8
-\
t
e
e
e
nd
nd
il
Tfl
td
Id
Id
rIa
Id
I
{
I
=
. :.1, .t;nciard^ibcuses on proportional relationships that can be represented as tables, graphs, equations, diagrams, and verbal
:;:: riptions. Str-rdents have already seen tables, grap!!, and verbal descriptions. The ulnifrate o., a graph is lhe point where x = l.
r:-r an equation. it is the slope represented by the coefficient, m, in the formula T = mx + b. The terris injt rate,ionstant of
:-: Dortionalih’. and slope are equivalent. Note that students are only required to read and interpret equitions in this standard.
-I-1
hI-{
b
I
AD
Facilitate a discussion about representations of
proportional relationships using a real-world scenario.
For erample, beginning with the verbal description: Mark
\\ as looking to fertilize his lawn, which is 172 rq. ft. He
re ad the packages of 5 diflerent fertili zer bags to see how
much should be used. Brg A stated 2 ounces per 4 square
feet, Brg B stated 4 ounces per B square feet, Brg C stated
1 .5 ounces per 3 square feet, and Brg D stated 6 ounces
per 12 sqr-rare feet. Are these rates proportional? If yes,
r,r’hat is the unit rate? How much fertilizer does Mark need
for his lawn?
Using a real-world context have students determine if the
relationship is proportional using graphs and/or tables. If it
is proportional, facilitate a discussion with the class on the
unit rate.
Share a verbal description of a proporiional relationship
and ask students to interpret it with a diagram such as
bars. Encourage students to write how they interpreted the
proportional relationship.
Introduce equations as a statement of the proportional
relationship. For the fertilizer story the equation is f – 2r,
where f is the amount of fertilizer needed and z is the size
of the lawn in square feet.
Provide students with a real-world proportional relationship
expressed in a verbal description, graph, table, and equation.
Challenge students to work with a partner to compare how
the unit rate is expressed in each representation. Share
student discoveries in alarge class discussion.
Finding the unit rate from a graph can be confusing. Some students
It is helpful to have a familiar unit rate students can recite such as I
in a coordinate pair, is the I and the y is the unit rate.
o Modei proportional relationships several different ways.
Translate a proportional relationship from a verbal
description into a diagram and explain in writing how the
diagram shows a proportional relationship.
Determine the unit rate from equations, graphs, tables,
diagrams, and verbal descriptions of proportional
relationships.
Discover that the unit rate (constant of proportionality) is
the numerical coefficient in the equation of a proportional
relationship.
cannot remember if the
CD for $ I 1.99 that helps
unit rate is the (1, y) or (x,1) point.
them remember the x, which is first
hrl
–JI.
ht
Tt
_-_-a
bE Part 1 Ratios and Proportional Relationships 21
, , ‘i:l;: r’i:’r, i-:. i_,ri:.r’!r’:r’
.. :, :
In the previous standard students read equations to find the unit rates. In this standard students are given verbal descriptions of
proportional relationships ,nJ ,r” .*p..i.d to
“r.rt.-
iil”l;;ii”;t in the form ! = mx: For example’ in Town C if you are caught
speeding, you receive , tr^in. ti.t.t. th” p”nrlty i, $25 for.u.ty $19 o19r the speed limit’ What is the dquation it p represents
the penalty r.,d. ,”pr”rJ’t’r-th. “r*U.t
if *l.t over the speed limit? The equation is ‘p =25*’
-ft
–
H
d provide students with real-world proportional reasoning
problems. Ask students to represent the stories as tables
,r,d graphs. Using the stud.tttg.tterated graphs and tables,
.r.ri. equatio* Ihrt model the proportional relationship.
provide students with real-world proportional relationship
problems presented as tables, graphs, and verbal
des.riptions. Have student, *rit.-equations to model those
relationships. Provide opportunities for students to write about how they
create equations that model Proportional relationships.
Some suggestions are exit slips, entrance slips, letters, and
journals.
Some students confuse the variables
letters closely representing what the
Model proportionai relationships presented as tables, r’erbal
descripliot t, and graphs in equation form’
]ustify in writing the reasoning used in creating an eg-uation agiven prop”ortional relationship expressed verbally.
in equations when they try to expressthe ?roportional relationship. It can be helpful to use
vrriaile, stand for such as’using ffor fertilizer instead ofx’
ilI-
-Ir
-T-
-hr \ 22 The common core Mathematics companion: The standards Decoded’ Grades 5-8 \ = -l, -rIt = t =l La LIIt An example of a proportional situation on a map suggests that I centimeter represents an actual distanc e of 4 . Explain the meaning of a point on a graph in the context of students at the school.”
o Discover that graphed proportional relationships are straight is: The scale Note the points (0,0) and (1,4). The 4 is the unit rate or slope of the line for the equation d = 4c, where d is total distance and c is Present students with a verbal description of a proportional Have students comp are graphs that show proportional o Focus on points (0,0) and (1, r), the origin and the unit . [Jse points that are not whole numbers and points where When finding points on a line that represents a real-world proportional relationship, students may think that the line stops at the Part 1 Ratios and Proportional Relationships 23
-1 f’
In this standard students solve problems involving proportional relationships. Students set up and
multiplication. For example : “Directions to make a tablecloth call for 1 *rO of ribbon for etery 2
1 Focus time on the vocabulary for this standard. Paper
foldables, word walls, graphic organizers, using words
in context, and writing stories all give students a chance
to clarify the meaning of these terms, which they may
encounter in daily life but not fully understand. Bring in the vocabulary. Vocabulary should include simple interest,
tax, tip/gratuity, discount, commission, fees, sale, markup, [Jse cross-multiplication to solve problems involving Begin with single-step problems and move to multi-step examples such as finding sales online, in print media, and
on TV.
Ask students to write problems that can be solved with Introduce students to percent increase/decrease and
percent error problems. Encourage students, through solve proportions using cross- yards of fabric. If you increase
To cross-rnultiply,
Explore use of the vocabulary words in this standard by
finding examples in the media and explain how they are
used in each situation.
Solve problems involving proportions using
cross-multipl ication.
Solve problems involving percent error and percent IJse the structure of percent error and percent increase/
decrease problems to explain how the formulas for these
concepts are similar.
t ]=z* percent increase/decrease, discounts, fees, markups, markdowns, discount, sales, and/or original prices.
To calculate a percent increase from 2 to 10, find the difference between the two numbers, in this case, 10 – 2 = 8. Take the
difference, 8, and divide by the original number: I = +. H,nultiply the quotient by 100: 4 x 100 = 400%.
Students may have misconceptions about the vocabulary commonly used in the media such as sale, discount, and tax. It is ind-ividuals with difficulties with particular words, use graphic organizers such as the Frayer model (see Reproducible 2). Acting
out situations can help students remember certain steps. For example, acting out shopping for a pair of tennis shoes and a tennis
racket and paying tax at the register will help students remember that tax is calculated on the cost of the total bill where the items
bought need to be added up before tax is calculated.
h.–
24 The Common Core Mathematics Companion: The Standards Decoded, Grades 6-8 tIIt-El-EI- Hl-HII \s
7.RP.A
::: cs and Proportional Relationships
I -ster A: Analyze proportional relationships and use them to solve real-world and mathematical problems.
lJse proportional relationships to sobe multi-step ratio and pur|urt problems.Examples: SFMP I. Make sense of problems and persevere in solving them.
Students solve multi-step problems involving percents.
SFMP 6. Attend to precision.
Students check answers to see ifthey are reasonable.
Students demonstrate understanding of the vocabulary tax and tip (gratuity) while solving multi-step problems.
Materials: paper and pencil, copies of restaurant menus
Sample Actittity:
o Provide groups of students with a menu and the following problem:
Route 15 Lunch Market Pulled Pork Sandwich $+.qg
Hamburger $:.:g Hot Dog $t.gg
Add fries and a 12-oz. Image courtesy of clipart.com.
Your group decides to keep working through lunch today so you will order lunch from the Route l5 Lunch Part 1 Ratios and Proportional Relationships 25
^a 99′ Stop Here
has r6-oz.’-ariw rris r “rg . which drink cosrs the least per ounce?” A;i;; students to create ratio and rate reasoning examples to comPare and solve real-world problems’ Students could use newspapers, store ads, or online ads to find th” .*I”-rpf.t ,r,a *Ltf the comparisons’ Ask students to use ,.rto”i;r to determine the better buys’ . Explore finding a percent of a quanlity as a rate per 100 such as 4A%of a quantity means # times the quantity’ NotingthataPercentisarateperl00,modelhowa Ask students to plot the points,o” 1 and draw conclisions ,bo,rt what is happening with the pr”Ui.* ,bou.. Students should reason that for every one movement to the right on the x-axis, the y-axis increases to ‘ th” Y-r*is decreases bY I 5 ‘ 10 The common core Mathematics companion: The Standards Decoded, Grades 6-8 3456 e -{ Td ; =t:::.: : i: l. repre sented with a hundreds grid by -: – u:rxrE a pe rcent w’heel (see Reproducible 1) or use double o Solr.e problems involving finding the whole, given . Examine the process of how to use ratio reasoning to . I foot Then multiply lZ-ilches x + = 60 inches. Create and interpret a table of equivalent ratios. Plot pairs of values from a table to a coordinate plane. Use a table to compare ratios and find missing values using Explain the difference betwden a ratio and a unit rate. [Jnderstand that rate problems compare two different units, Solve real-world problems using ratios and rates. Reason to determine the better buy. Write a percent as a rate over 100, including percents Find the percent of a number using rate methods. Represent the relationship of part to whole to describe Convert units by multiplication or division. ild Then 60 inches x z’54 cm – 15 2.4 cm. (I\ote that conversions can be made between units within a medsurement system such as inches to feet or between systems such as miles to centimeters.) Allow students to talk with each other and their teacher to Focus on the following vocabulary terms: ratios, rdtes, Provide cyclical, distributed practice over time to Some sixth graders misunderstand and believe that a percent is always a natural number less than or equal to 100. To help with T Efl– = EI 7,’nt t’ 7.RP.A* 7.RP.A.l: Compute unit rates associated with ratios of fractions, including ratios of lengths, areas and other quantities measured in like or different :units. For example, if a person walks L mile in each ! hour, compute the unit rate as the complex fraction Z mibs per hour, equi’talently 2 miles per 7.RP.A.2: Recognize and represent proportional relationships between quantities. a. Decide whether two quantities are in a proportional relationship, e.g., by testing for equivalent straight line through the origin. b. Identify the constant of proportionality (unit rate) in tables, graphs, equations, diagrams, and c. Represent proportional relationships by equations. For example, if total cost t is proportional and the number of items can be expressed as t = pn. d. Explain what a point (x, y) on the graph of a proportional relationship means in terms of the 7.RP.A.3: Use proportional relationships to solve multi-step ratio and percent problems. Examples: oMajor cluster Cluster A: Analyze proportional relationships and use them to solve real-world and mathematical problems. Grade 7 Overview These standards extend what students learned in Grade 6 about ratios to analyzingproportions and proportional relationships. world scenarios such as interest, tax, shopping sales, and so on. ‘ Standards for Mathematical Practice SFMP 1. Make sense of problems and persevere in solving them. Students solve multi-step ratio and real-world percent problems. SFMP 3. Construct viable arguments and critique the reasoning of others. Students recognize proportional relationships from non-proportional ones and discuss their reasoning with others. E -F16 The Common Core Mathematics Companion: The Standards Decoded, Grades 5-8 r ir)s€,ts$red fn ifke *r csrnpl*x fractinn assocrated with ratios of fractions, including ratios of lengths, areas and olher q,uantities different units. For example, if a person walks J mile in each ] hour, compute the unit rate at the24 This standard focuses on computing unit rates using ratios of fractions known as complex fractions. In a complex fraction, the -L numerator, denominator, or both are fractions. In the standard, { is an example of a complex fraction. Complex fractions can ,L+ thought of ar ] + { . Applications include situations where the 4 What th* TfiACI'{ER d*es: to unit rates with whole numbers from Grade 6. o Treat complex fractions as division of fractions. . Set up error analysis scenarios where students can identify example, Homer calculated that if a person walks + mile every + hour, the unit rate is 2 miles. However, Homer,4 Homer why 2 miles is not the correct answer. o Provide opportunities for students to compute the unit rates in real-world problems. What the STUDENTS do: whole numbers is the same concept as unit rates with ratios . Compute unit rates in real-world problems that involve . In writing, explain the errors that can be made when Sometimes the format of a complex I + I . Discuss how the divisionZ4 Addressing Student Misconceptions and Common Errors It is not uncommon to find seventh-grade students who are not fluent with fraction division. fraction confuses them when they are used to seeing fraction division written horizontally as bar in the complex fraction means the same as the symbol +. For students having difficulty underStanding unit rate and those having trouble with different units such as miles per hour, pictures and diagrams may help. Use the example from this standard: lf a person walks L mile in each \ hour, compute the unit’ rate as the complex fraction t miles per hour, equivalently 2 miles per hour. Model with a diagram as shown. The bar represents I hour broken into ] hour rlg.n.r,t . mile I I I I mile mile mile From this diagram, students can see that the word problem is showing mile everv hour.I I L L- LHIHIH E Sections a-d of 7.RP.A.2 break down the standard to give guidance on ways to recognize and represent proportional relationships. This standard emphasizes hryo metliods for deciding whether a proportional relationship exists. One method is to use equivalent # of people in a room # of hands in the room The other method is to graph the relationship on a coordinate plane and observe whether the graph is a straight line that goes r2345 Explore proportional reasoning scenarios with students Ask students to write their own examples and non-examples Discuss equivalent ratios with the students. Ask them fractions. Display the pairs as the students suggest them in the form t=i, where b + }and d * 0. Define two equivalent ratios as a proportion. Pose examples of proportions written with the quantities in fuk students to explain how they know is not a correct . Craph two ratios on a coordinate plane from a proportional .(3, 18) Pose the task to students: Select other points on the graphed 3 1 15 5 r 5 E:’ O’T=T O’ 7:E I I I t”l I I Ii ‘(1’ 6) I I I I <=--- I
-_-+,.
I
I
7 15or;:TI)
15 _ 2T7
Provide examples of equivalent and non-equivalent ratios
to students for them to test with a table to decide if they are
proportions. Conversely, present students with a table for a
context and ask them to determine if all of the entries in the
table are proportional.
Pa rt 1 Ratios a nd Proportiona I Relationsh ips 1 9
I
What the STUDENTS do:
. Sort real-world exampl.Ss of proportional relationships from
non-examples. Students can create their own examples
to demonstrate that they understand the concept of
proportional relationships when there is a context attached.
o Communicate orally and/or in writing that a proportion is a
statement of two equivalent ratios. Students apply what they
know about equivalent fractions to equivalent ratios"
o Model proportional relationships by creating tables;
determine if a proportional relationship exists from a given
table.
Model relationships on graphs to determine if they are
proportional.
Test their hypotheses about whether a proportional
relationship exists between any two points on the lines
graphed. Students may draw the conclusion that all points
on the line are proportional toall other points on the line
by relying on tables, verbal statements, or logic al arguments
to draw the conclusion q
E
E
l
E
c
d
T
E
Addressing Student Misconceptions and Common Errors
While graphing, students may need to be reminded that the same types of quantities need to be graphed on the same axis. For
example, when checking to determine if I0 cans of soda for $2 is proportional to 50 cans of soda for $10, the cans of soda must
both be represented on the same axis and the dollar amounts must be on the other axis. Ensure students are using graph paper or
graphing calculators for all graphing. Remind them to label the axes.
i
\
I
20 The Common Core Mathematics Companion: The Standards Decoded, Grades 5-8
-\
t
e
e
e
nd
nd
il
Tfl
td
Id
Id
rIa
Id
I
{
I
=
. :.1, .t;nciard^ibcuses on proportional relationships that can be represented as tables, graphs, equations, diagrams, and verbal
:;:: riptions. Str-rdents have already seen tables, grap!!, and verbal descriptions. The ulnifrate o., a graph is lhe point where x = l.
r:-r an equation. it is the slope represented by the coefficient, m, in the formula T = mx + b. The terris injt rate,ionstant of
:-: Dortionalih'. and slope are equivalent. Note that students are only required to read and interpret equitions in this standard.
-I-1
hI-{
b
I
AD
Facilitate a discussion about representations of
proportional relationships using a real-world scenario.
For erample, beginning with the verbal description: Mark
\\ as looking to fertilize his lawn, which is 172 rq. ft. He
re ad the packages of 5 diflerent fertili zer bags to see how
much should be used. Brg A stated 2 ounces per 4 square
feet, Brg B stated 4 ounces per B square feet, Brg C stated
1 .5 ounces per 3 square feet, and Brg D stated 6 ounces
per 12 sqr-rare feet. Are these rates proportional? If yes,
r,r'hat is the unit rate? How much fertilizer does Mark need
for his lawn?
Using a real-world context have students determine if the
relationship is proportional using graphs and/or tables. If it
is proportional, facilitate a discussion with the class on the
unit rate.
Share a verbal description of a proporiional relationship
and ask students to interpret it with a diagram such as
bars. Encourage students to write how they interpreted the
proportional relationship.
Introduce equations as a statement of the proportional
relationship. For the fertilizer story the equation is f - 2r,
where f is the amount of fertilizer needed and z is the size
of the lawn in square feet.
Provide students with a real-world proportional relationship
expressed in a verbal description, graph, table, and equation.
Challenge students to work with a partner to compare how
the unit rate is expressed in each representation. Share
student discoveries in alarge class discussion.
Finding the unit rate from a graph can be confusing. Some students
It is helpful to have a familiar unit rate students can recite such as I
in a coordinate pair, is the I and the y is the unit rate.
o Modei proportional relationships several different ways.
Translate a proportional relationship from a verbal
description into a diagram and explain in writing how the
diagram shows a proportional relationship.
Determine the unit rate from equations, graphs, tables,
diagrams, and verbal descriptions of proportional
relationships.
Discover that the unit rate (constant of proportionality) is
the numerical coefficient in the equation of a proportional
relationship.
cannot remember if the
CD for $ I 1.99 that helps
unit rate is the (1, y) or (x,1) point.
them remember the x, which is first
hrl
--JI.
ht
Tt
_-_-a
bE Part 1 Ratios and Proportional Relationships 21
, , 'i:l;: r'i:'r, i-:. i_,ri:.r'!r':r'
.. :, :
In the previous standard students read equations to find the unit rates. In this standard students are given verbal descriptions of
proportional relationships ,nJ ,r" .*p..i.d to
"r.rt.-
iil"l;;ii";t in the form ! = mx: For example' in Town C if you are caught
speeding, you receive , tr^in. ti.t.t. th" p"nrlty i, $25 for.u.ty $19 o19r the speed limit' What is the dquation it p represents
the penalty r.,d. ,"pr"rJ't'r-th. "r*U.t
if *l.t over the speed limit? The equation is 'p =25*'
-ft
-
H
d
-JHlJ*1
JId
La LIIt An example of a proportional situation on a map suggests that I centimeter represents an actual distanc e of 4 . Explain the meaning of a point on a graph in the context of students at the school.” o Discover that graphed proportional relationships are straight is: The scale Note the points (0,0) and (1,4). The 4 is the unit rate or slope of the line for the equation d = 4c, where d is total distance and c is Present students with a verbal description of a proportional Have students comp are graphs that show proportional o Focus on points (0,0) and (1, r), the origin and the unit . [Jse points that are not whole numbers and points where When finding points on a line that represents a real-world proportional relationship, students may think that the line stops at the Part 1 Ratios and Proportional Relationships 23 -1 f’ In this standard students solve problems involving proportional relationships. Students set up and multiplication. For example : “Directions to make a tablecloth call for 1 *rO of ribbon for etery 2 1 Focus time on the vocabulary for this standard. Paper foldables, word walls, graphic organizers, using words in context, and writing stories all give students a chance to clarify the meaning of these terms, which they may encounter in daily life but not fully understand. Bring in the vocabulary. Vocabulary should include simple interest, tax, tip/gratuity, discount, commission, fees, sale, markup, [Jse cross-multiplication to solve problems involving Begin with single-step problems and move to multi-step examples such as finding sales online, in print media, and on TV. Ask students to write problems that can be solved with Introduce students to percent increase/decrease and percent error problems. Encourage students, through solve proportions using cross- yards of fabric. If you increase To cross-rnultiply, Explore use of the vocabulary words in this standard by finding examples in the media and explain how they are used in each situation. Solve problems involving proportions using cross-multipl ication. Solve problems involving percent error and percent IJse the structure of percent error and percent increase/ decrease problems to explain how the formulas for these concepts are similar. t ]=z* percent increase/decrease, discounts, fees, markups, markdowns, discount, sales, and/or original prices. To calculate a percent increase from 2 to 10, find the difference between the two numbers, in this case, 10 – 2 = 8. Take the difference, 8, and divide by the original number: I = +. H,nultiply the quotient by 100: 4 x 100 = 400%. Students may have misconceptions about the vocabulary commonly used in the media such as sale, discount, and tax. It is ind-ividuals with difficulties with particular words, use graphic organizers such as the Frayer model (see Reproducible 2). Acting out situations can help students remember certain steps. For example, acting out shopping for a pair of tennis shoes and a tennis racket and paying tax at the register will help students remember that tax is calculated on the cost of the total bill where the items bought need to be added up before tax is calculated. h.– 24 The Common Core Mathematics Companion: The Standards Decoded, Grades 6-8 tIIt-El-EI- Hl-HII \s 7.RP.A ::: cs and Proportional Relationships I -ster A: Analyze proportional relationships and use them to solve real-world and mathematical problems. lJse proportional relationships to sobe multi-step ratio and pur|urt problems.Examples: SFMP I. Make sense of problems and persevere in solving them. Students solve multi-step problems involving percents. SFMP 6. Attend to precision. Students check answers to see ifthey are reasonable. Students demonstrate understanding of the vocabulary tax and tip (gratuity) while solving multi-step problems. Materials: paper and pencil, copies of restaurant menus Sample Actittity: o Provide groups of students with a menu and the following problem: Route 15 Lunch Market Pulled Pork Sandwich $+.qg Hamburger $:.:g Hot Dog $t.gg Add fries and a 12-oz. Image courtesy of clipart.com. Your group decides to keep working through lunch today so you will order lunch from the Route l5 Lunch Part 1 Ratios and Proportional Relationships 25 ^a
-JHlJ*1
JId
‘for
aT-
E
b
J
I=
E
ts
bi
-ha
lr
tt
–
IIt
IIa
Ia
II
–
I
)
–
=
-1
>d
_J
t
tt-II
!-
=i
kilometers. The map distance between
rePresented as:
B centimeters. What is the actual distance? The graph of this relationship is
the situation. Students should be able to explain examples
with words such as, “Point (5, 7) is the point that represents
5 health bars for $7 .00″ or ” (7, 10) represents the unit rate
(constant of proportionality), meaning 1 teacher for every 10
lines.
two towns is
the number of centimeters.
relationship and build the graphical representation with the
students. Be sure students give input on the labels for the
x- and /-axes. Facilitate a discussion about the graph with
students asking them for the meaning of individual points
and asking students to justify their responses.
relationships and talk to a partner about what they notice.
rate, respectively.
students need to estimate the coordinates.
origin. The teacher should show that the line continues into Quadrant III but that the points are not appropriate for the real-
world situation. For example, in g proportional relationship between the number of teachers and the number of students at a
grade level, it does not make sense to have -3 teachers.
the amount of fabric used to 3 yards, hoi,, much ibbon will be needed?” The proportio ” ” + = ; .
items familiar to students such as tennis shoes, a six-pack of
soda, and so on and use them to model situations that use
markdowil, and original price.
proportional relationships. Use numbers in your problems
that do not lend themselves easily to mental arithmetic.
using a wide variety of contexts. Make use of everyday
setting up proportions prompted by media ads.
questioning, to discover the similarities among the formulas
for these concepts.
o
increase/decrease.
Problems for this standard should be multi-step and include contexts with simple interest, tax, tips, commissions, percent error,
important to discuss what students already know about these words in order to correct any pre-existing misconceptigns, For
–
ft-
ts
I
–
aI
EI!
=ts
L
E
E
I
simple interest, tax, markups and markdowns, gratuities and commissions, fees, percent increase and decriase,
percent error.
Take-Out Menu
Cheesebu rger $:.gg
Chicken Sandwich $q.qg
Fish Sandwich $q.+g
drink for $1.99 more!
Market.
(continued)
coordinate plane
15x. Also, for every one movement to the left on the x-axis’
Number of GDs
e
-{
IId
IIi
-,. -:.. ; .:,l,-, units. Have students write this as a fraction
. a ie cinral r0.40), and a percent $A%). Consider
nunrber lines and tape diagrams in which the whole is 100
to find the rate per hundred.
a part and the percent such as, “What is 40% of 60?
80% of what number is 300? Or 50 is 30% of
what number?”
convert measurement units such as, “How mdny centimeters
are in 5 feet?” Use the information that I inch = /.54 cm.
Represent the conversion of 12 inches : I ft as a conversion
lactor in ratio form, 12. ilches
I foot I
ratios.
such as revolutions per minute.
greater than 100 and less than I .
percents using models.
T4
I inch
make sense of what they are learning.
unit rdtes, equivalent ratios, percents, ratio tables, and tape
diagrams.
continually practice unit rate problems.
this misconception, provide examples of percent amounts that are greater than 100% and percent amounts that are less than I %.
Try using a percent wheel for developing this understanding. See Reproducible 1.
‘It
EI
E1
ts
{
h
!=
h,t
b:il
b
E
ET
i,E[-r Part 1 Ratios and Proportional Relationships 1’l
t’-Lnour. 4
ratios in a table or graphing on a coordinate plane and observing whether the graph is a
verbal descriptions of proportional relationships.
to the number n of items purchased dt o constdnt pice p, the relationship between the total cost
situation, with special attention to the points (0, 0) and (1, r) where r is the unit rate.
simple interest, tax, markups and markdowns, gratuities and commissions, fees, percent increase and
decrease, percent enor.
Students calculate unit rates with complex fractions and move to recognizing and representing proportional relationships in
equations and on graphs. These skills and understandings are used to solve multi-step ratio and percent problems involving real-
t
tr
E
t
E
t
t
E
E
E
E
E
t
E
E
E
tr
E
Eh
{crnpute r”l,tif raies
:1
?
e
milesperhouLequivalently2milesperhour……i-
be interpreted as division statements. For example, ! “^nbe
quantities are measured in different units such as miles per hour, pounds per square foot, feet per second, and so on.
o Explore unit rates with ratios of fractions and compare them
errors in computing unit rates with complex fractions. For
2
made an error. F ind his error, correct it, and explain to
o Discover that the structure of computing unit rates with
of fractions.
complex fractions.
computing unit rates with complex fractions and unlike
units.
_L- 2 4
4
1
1
1
1
J
1
z
18 The Common Core Mathematics Companion: The Standards Decoded, Grades 6-8
Fb.5
EL-
I-J
LI
L
L-)
ratios in a table. Ifthe ratios are equivalent, then you have a proportional relationship such as:
through the origin. Note that computation using cross-multiplication is not a part of this standird.
2+68?
to be sure they understand the meaning of proportional
relationships in context before using the tables or graphs.
While some number combinations may be proportional,
the real-world example attached to the numbers may
not be. [Jse examples and non-examples for students to
identify and compare. An example is: “2 music downloads
cost $1.98; therefore, 4 music downloads cost $3.96.” A
non-example ts: “Three boys cdn run d mile in 10 minutes;
therefore, 6 boys cdn run d mile in 20 minutes.”
of proportional relationships. Student work can be shared
and discussed.
to suggest some equivalent pairs. Relate to equivalent
different positions. Encourage students to decide if there
is more than one correct way to set up a proportion. For
exampl e: “Set up d proportion showing that 3 out of
15 students are girls is the sdme ratio as I out of 5 students
are girls.”
proportion for the example.
scenario and look for a straight line that goes thrrough the
origin to determine if the two ratios are proportional. For
exampl e: “Mdria sells necklaces and makes a profit of $6 for
each necklace. How much monq) does she make for selling
3 neckldces?”
line and determine if they are also proportional.
I
_J
t
tt-II
!-
=i
kilometers. The map distance between
rePresented as:
B centimeters. What is the actual distance? The graph of this relationship is
the situation. Students should be able to explain examples
with words such as, “Point (5, 7) is the point that represents
5 health bars for $7 .00″ or ” (7, 10) represents the unit rate
(constant of proportionality), meaning 1 teacher for every 10
lines.
two towns is
the number of centimeters.
relationship and build the graphical representation with the
students. Be sure students give input on the labels for the
x- and /-axes. Facilitate a discussion about the graph with
students asking them for the meaning of individual points
and asking students to justify their responses.
relationships and talk to a partner about what they notice.
rate, respectively.
students need to estimate the coordinates.
origin. The teacher should show that the line continues into Quadrant III but that the points are not appropriate for the real-
world situation. For example, in g proportional relationship between the number of teachers and the number of students at a
grade level, it does not make sense to have -3 teachers.
the amount of fabric used to 3 yards, hoi,, much ibbon will be needed?” The proportio ” ” + = ; .
items familiar to students such as tennis shoes, a six-pack of
soda, and so on and use them to model situations that use
markdowil, and original price.
proportional relationships. Use numbers in your problems
that do not lend themselves easily to mental arithmetic.
using a wide variety of contexts. Make use of everyday
setting up proportions prompted by media ads.
questioning, to discover the similarities among the formulas
for these concepts.
o
increase/decrease.
Problems for this standard should be multi-step and include contexts with simple interest, tax, tips, commissions, percent error,
important to discuss what students already know about these words in order to correct any pre-existing misconceptigns, For
–
ft-
ts
I
–
aI
EI!
=ts
L
E
E
I
simple interest, tax, markups and markdowns, gratuities and commissions, fees, percent increase and decriase,
percent error.
Take-Out Menu
Cheesebu rger $:.gg
Chicken Sandwich $q.qg
Fish Sandwich $q.+g
drink for $1.99 more!
Market.
Essay Writing Service Features
Our Experience
No matter how complex your assignment is, we can find the right professional for your specific task. Achiever Papers is an essay writing company that hires only the smartest minds to help you with your projects. Our expertise allows us to provide students with high-quality academic writing, editing & proofreading services.
Free Features
Free revision policy
$10Free bibliography & reference
$8Free title page
$8Free formatting
$8How Our Dissertation Writing Service Works
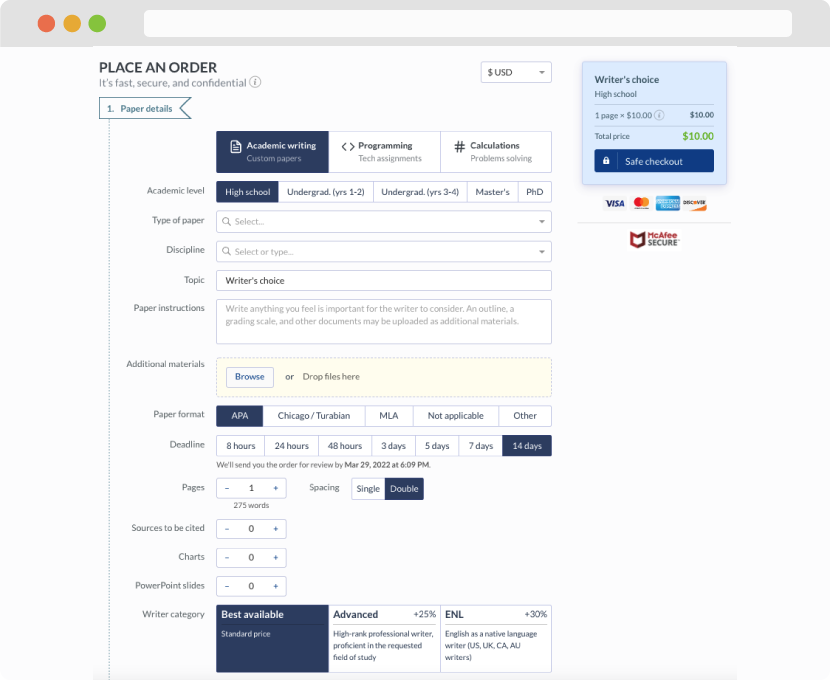
First, you will need to complete an order form. It's not difficult but, if anything is unclear, you may always chat with us so that we can guide you through it. On the order form, you will need to include some basic information concerning your order: subject, topic, number of pages, etc. We also encourage our clients to upload any relevant information or sources that will help.
Complete the order form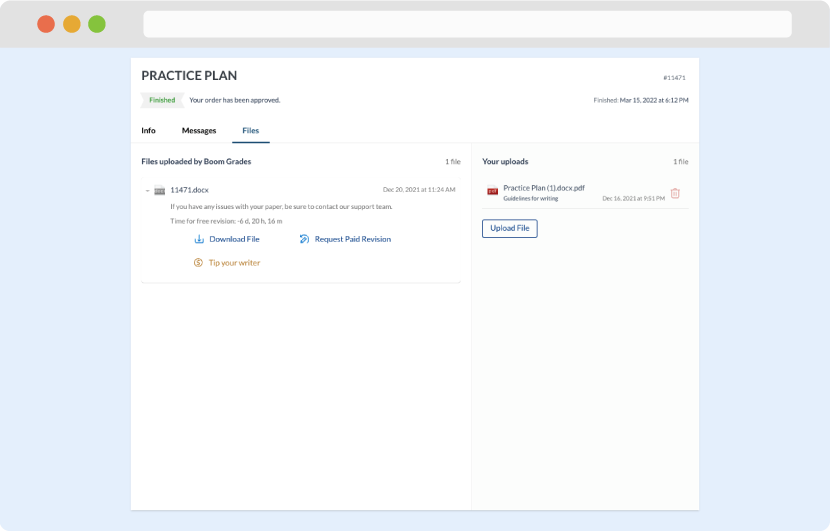
Once we have all the information and instructions that we need, we select the most suitable writer for your assignment. While everything seems to be clear, the writer, who has complete knowledge of the subject, may need clarification from you. It is at that point that you would receive a call or email from us.
Writer’s assignment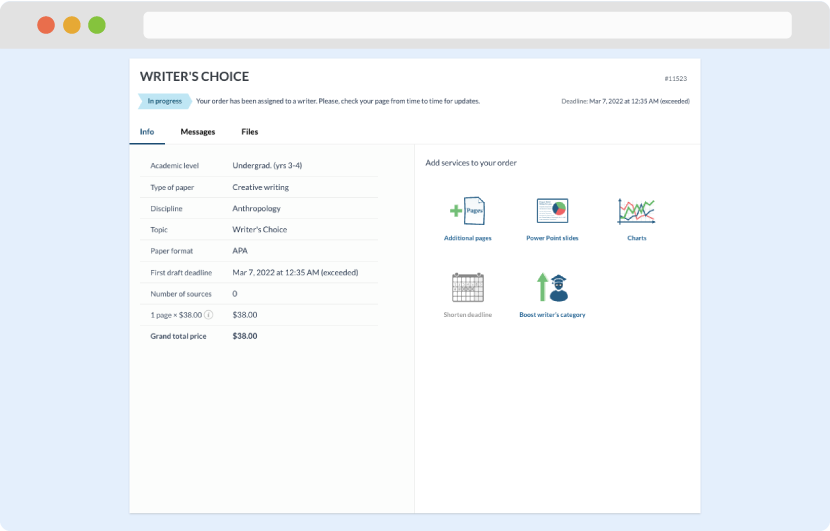
As soon as the writer has finished, it will be delivered both to the website and to your email address so that you will not miss it. If your deadline is close at hand, we will place a call to you to make sure that you receive the paper on time.
Completing the order and download